Energy stored in a capacitor

Objective :
To derive the expression for energy stored in a capacitor.


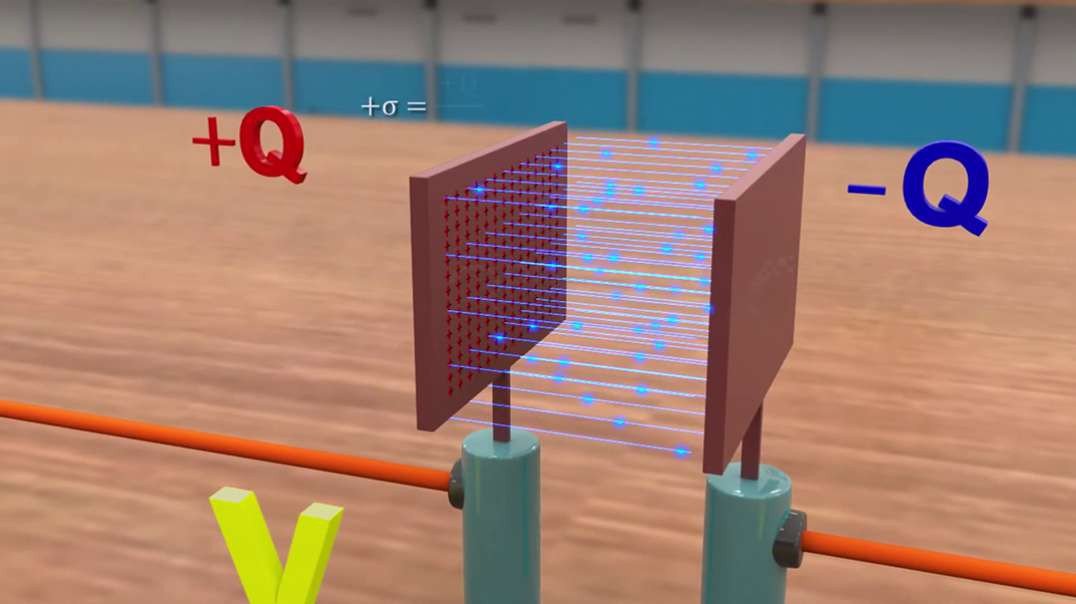
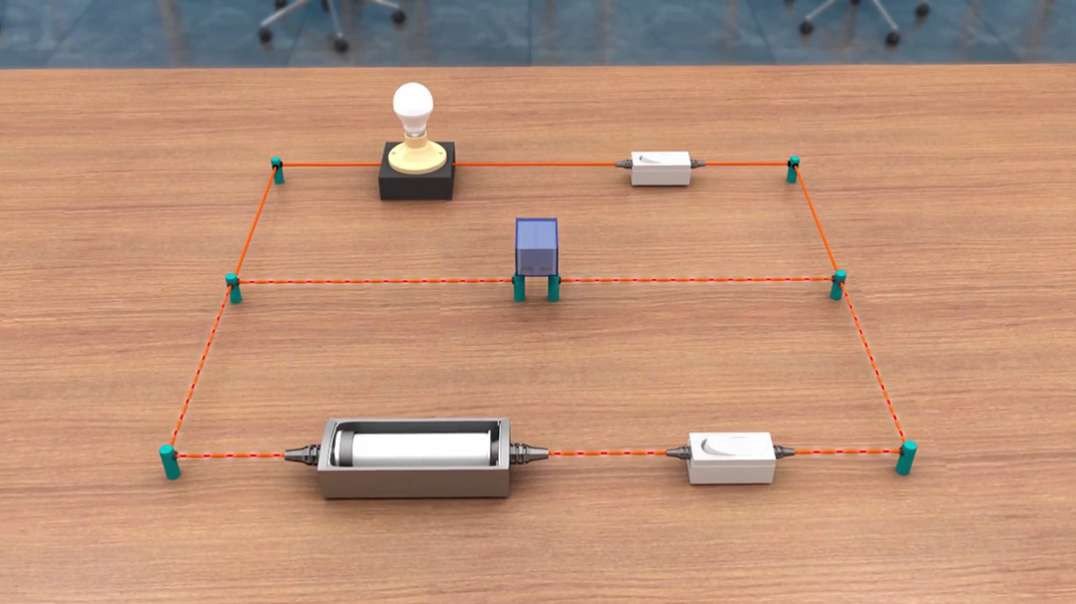
Consider a parallel plate capacitor, whose plates of area ‘A’ each, are kept at a distance ‘d’ from each other.

Capacitance, ‘C’, of the capacitor is therefore C=∈0Ad .


Let a potential difference, ‘v’, be applied across its plates.


The charge, ‘q’, stored on the capacitor will then be equal to ‘cv’.

If the potential of the positive plate is V+,

and that of the negative plate is v – , the difference in potential, V+ – V – = V.

Let us find the potential energy of each plate from the expression for potential energy of continuous charge distribution.


Electric potential energy is U=12∫0qV(dq).


Consider a small charge, ‘dq’, on the positive plate.

It is at a potential, V+.

V+ is constant for the charge on the positive plate.

Hence, potential energy of the positive plate is, 12V+∫0qdq , over the entire charge on the positive plate.

So, the potential energy of the first plate is, half Q, V+.


Similarly, the negative plate is at a uniform potential, V–.

So, the negative plate has potential energy given by -12qV .


Total potential energy of the capacitor = 12qV+-12qV-

But, V+ – V– , is V.

Hence, potential energy of the capacitor is 12qV.


Substituting Q with C V, we get potential energy to be equal to 12CV2.


Substituting V with Q by C, we get energy to be 12q2C.

Consider the field, E, between the capacitor plates; it is uniform between the plates


So, the potential difference, V, across the plates is equal to, E בd’.

Substituting, ‘C’ and ‘V’ in the energy expression, we have

U=12 ∈0(Ad)E2 where

A d is the volume of the space occupied by electric field, ‘E’.

Bringing the volume term to the left side of the equation we get, energy per unit volume

This ratio is nothing but energy density, given by 12 ∈0E2 .


In electrostatics, we can either consider energy to be stored in the charge distribution or in the field.


Both points of view are valid and refer to the same energy


Summary :


Energy stored in the capacitor U=12qV=12CV2=12q2c.


Energy density in the capacitor =12 ∈0 E2 .